Image Source.
Gl 436 b orbits its parent star in a short 2.64 days, and the discovery of transits indicates that its physical properties are quite similar to Neptune. The theoretical expectation is thus completely clear cut. “That orbit is circular, Son. Tidal dissipation has long since damped out that eccentricity.”
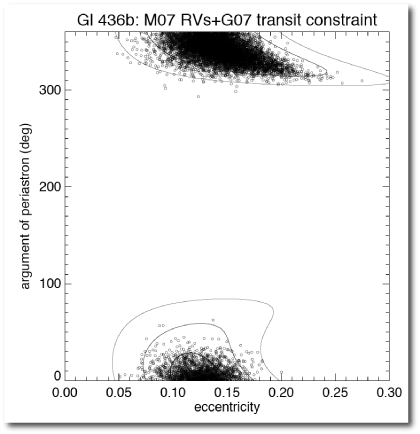
The data, however, stubbornly insist otherwise. When I do a one-planet fit to the radial velocities (incorporating the constraint on the mean anomaly imposed by Gillon et al.’s observation of the transit midpoint) then the distribution of bootstrap fits indicates e~0.13 +/- 0.03:
[Note: Stefano and Eugenio have been cranking away on the downloadable console code base, and the current beta-test version on the backend now contains a slew of new features, including a revved-up Hermite integrator and the ability to incorporate transit timing observations into the orbital fits. The user interface has been completely overhauled in order to maintain usability with the rapidly expanding feature set. We’ll be putting up some posts very soon that demo all this bling. In the interim, though, I definitely recommend downloading a copy and taking it for a test-drive.]
The latest console version.
In this post from last week, I looked at the possibility that gl 436 b’s eccentricity is being maintained by as-yet unpublished planets. There’s a hint of a long-term trend in the data that indicates a large and distant companion.
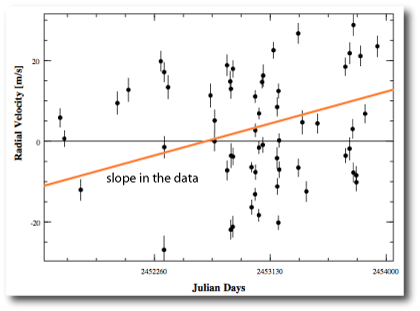
The lowest chi-square fit to th gj437_M07K data set (by user Schneidi) reduces the magnitude of the long-term trend by using a pair of planets on 53 and 399 day orbits.
In Schneidi’s fit, the bulk of the perturbation on planet b is provided by the 53-day plant “c” which also has close to a Neptune mass. In last week’s post, I looked at this model in gory detail. If the 53-day planet exists, and if its orbital plane is aligned for transits, then the transit will occur around June 7th.
For two planets like Gl 436 b and c, which aren’t in mean-motion resonance, and which aren’t on crossing orbits, the long-term evolution of the orbits is well-described by an approximation worked out by Laplace and Lagrange in the 1770s. In the Laplace-Lagrange theory, the gravitational interactions between a set of planets are assumed to be effective over a “secular” timescale that is much longer than the orbital periods of the planets themselves. The planets can thus be treated as flexible elliptical wires of varying mass density (highest near apoastron where the planets spend more time, and lowest near periastron where the least time is spent). The planets are able to trade eccentricity back and forth while keeping their semi-major axes fixed (orbital angular momentum is exchanged, but not orbital energy).
Last week, I was wondering whether the secular interchange of eccentricity could provide a mechanism for b to offload angular momentum as it tidally dissipates its orbital energy. If such a mechanism were effective, then it might explain why b’s orbit is still eccentric.
To look at this, I used a “double averaging” approximation to do a long-term numerical evolution of the 2-planet system in the presence of tidal damping. With this approach, one uses the Laplace-Lagrange theory to advance the system forward over a secular timestep of hundreds to thousands of years. After each secular timestep, one then applies tidal dissipation (modify semi-major axis and eccentricity so as to decrease the energy of planet b while conserving its angular momentum). Then one takes another secular timestep, etc. This approach should provide a reasonable picture of the orbital evolution so long as the secular time scale (thousands of years) is much shorter than the tidal evolution time scale (millions of years or more).
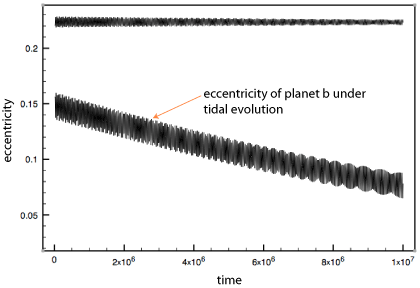
The answer is immediately clear. The presence of a 53-day planet “c” doesn’t stave off tidal circularization. In the graph above, I’ve assumed a Neptune-like tidal Q of 10,000 for b. The high-frequency secular exchange of angular momentum is of no use for maintaining b’s eccentricity. The orbit is circularized on an e-folding timescale of ~10 million years — much shorter than the current age of the star.
Guess I’m just not hip to where b’s scoring its e.

Hi Greg,
Bummer! I had such high hopes for the double averaging approach and in the Lagrange-Laplace (LL) secular theory. This is not good. According to your analysis we have a planet, i.e. Gl 436b that has a minimal eccentricity of e~0.13 +/- 0.03 and no way of explaining it.
Since there’s a hint of a long-term trend in the data that indicates a large and distant companion, maybe I was right in the beginning when I said Marcy et al need to go back to Keck and collect more data to see if there is indeed a massive and distant companion. I hate to tell you that I told you so, but….. I’m sorry it didn’t work out the way you wanted it too. I know you must be as bummed out as I am about this.
But, in the mean time, we have a planet that’s supposed to have an almost circular orbit, but in fact has an orbit with eccentricity ~0.13. Something in that system has staved off circularization, but I believe we need more data to find it. I don’t think there’s enough data to even establish an orbital period much less any other parameters.
I don’t know how priorities are set for allocation of precious telescope time on an observing run. But, if we don’t try to gain more data for this system in an attempt to explain the “e” of Gl 436 b, then I’m afraid the Swiss are going to again beat us to the punch. And, I for one am sick of getting scooped by the Swiss. No offense intended toward our Swiss bloggers. I’m just simply speaking my mind. And, with all of the fits that I’ve done for these systems, I think I’ve earned the right.
Best regards,
Eric
In keeping with the perturbation issues, I wonder what you guys make of HD 73526 B&C.
They have twice the mass of Jup, are at about 0.65AU & 1.04AU AND have Eccentricities of 0.39 & 0.4 respectively !!!!
Hmm! there appears to be a descrepancy on e as reported in exoplanet.org & the Exoplanet catalog. I would imagine the formers value as I reported is more authentic.
HD 73526 b and c seem to be participating in a 2:1 mean-motion resonance, which is keeping the system stable over the long term. When one does fits to the radial velocities, there’s quite a bit of uncertainty in the osculating eccentricities, so it’s not surprising that the various catalogs are describing different fits to the data.
I wrote an oklo.org post about the HD 73526 system in March 2006. It includes a neat animation of the orbits over a several thousand year time frame.
You may also want to check out the analysis that we did in the discovery paper. The preprint(Tinney et al. 2006) is on astro-ph.
Greg-
Looking at Maness et al., they seem to think that with Q=100000, the tidal damping timescale is about 2 billion years, i.e. in 2 billion years the eccentricity will drop by a factor of e=2.74 (the base of the natural logarithm). Given a 11 billion year age, the current eccentricity is still rather high, but one solution is that this hot Neptune has a Q of more than a few hundred thousand. (Given how little we actually understand about the tidal Q parameter, this doesn’t seem unreasonable.)
But suppose Q is low. If secular effects can’t do it, then I bet we’re looking at a resonant system. Looking at a portion of the RV curve for GJ 876, it would be easy to confuse a single planet with a long trend to two interacting resonant planets. Supposing it is in some resonance leads to two questions: 1) with the correct fitting, what are the actual eccentricities of the objects? (For this, more data is strongly warranted.) 2) Can this resonant system maintain eccentricies for long times? Tides on the inner planet drain angular momentum from the whole resonant system so a resonance still might not be strong enough.
Just some thoughts,
Darin
Darin,
The 2 Gyr timescale in the Maness paper hinges partly on their adoption of a radius of 22^(1/3) Earth Radii=17,700km for 436b. The transit measurement indicates that the real radius its actually ~1.4 times larger than this. Because of the inverse 5th-power scaling, this corresponds to a factor of 5.6 in timescale. So a 2 billion year timescale would actually require a Q more in the range of 500,000. This seems pretty high.
Resonance is an interesting idea. Eugenio had some very compelling fits along those lines to the RV data alone. Now that the console can incorporate the transit constraints, it’ll be interesting to revisit this possibility.
Greg
Hi Darin and Greg,
I suppose resonance is still a possibility but a remote one at best, and I’m not holding my breath. A Q in the range of 500,000 does seem unlikely, but that was an interesting thought, Darin.
But, one thing that I absolutely I agree with you on Darin, is that regardless of what the case might be, more data is “strongly warranted”. But, I very much doubt that that’s going to happen.
Eric
I’m going to be very blunt, and this will be the last thing I have to say about gj437_M07K. Now, I don’t presume to have the expertise nor the understanding that Greg, Eugenio and Stefano have. But, I have worked with these systems for quite some time now and have done a lot of reading on resonance and secular perturbation. And, I believe that I have a pretty good understanding of what is going on in most of these systems.
I don’t believe that resonance is going to explain the eccentricity of gj437b. I do believe that there is a very massive brown dwarf out there, yet to be detected, that is causing secular perturbation. I also believe that in time the Swiss are going to find it, and that the U.S. and Systemic are both going to end up having egg on their respective faces. I hope I’m wrong, but I doubt it. And, that’s the last I have to say about gj437_M07K.
Eric
Typo Correction: I meant gj436_M07K and gj436b. Sorry for any confusion.
Eric
I see the bootstrap trials give an e of 0.13 +/- 0.03 in Greg’s fit, but I’m not sure that’s enough to convince me there has to be eccentricity. A circular orbit gives a chi square of 5.70 and an F-test between the circular fit and the best eccentric single planet fit gives a result of 1.0982 and a probability of 0.72.
Chris
Pingback: systemic - A hot hot Neptune