Vladimir Arnold, he of the A in KAM Theory, wrote a classic graduate text entitled Mathematical Methods of Celestial Mechanics. This, as one might imagine, is a book that is not exactly a storehouse of easy homework assignments. There are, however, a scattering of problems that offer insights while, at the same time, not actually requiring the tough-guy methods that are the text’s primary focus.
During his walk in outer space [as part of the Voskhod 2 Mission on 18 March 1965], the cosmonaut Alexey Arkhipovich Leonov threw the lens cap of his movie camera toward the Earth. Describe the motion of the lens cap with respect to the spacecraft, taking the velocity of the throw as 10 m/s. Neglect the asphericity of the Earth.

(Ria Novosti/Science Photo Library)
Leonov’s space walk tipped off a hair-rising adventure which began with his being nearly unable to re-enter the spacecraft, and ended with a frigid way-off-course landing in the Siberian Tiaga, all of which is covered in a recent BBC documentary.
One can hand-crank the problem by noting that the radially directed, \({\bf v}_{i}=10\,{\rm m\,s^{-1}}\), launch of the lens cap exerts no torque, so that \({\bf r}\times{\bf v}_{i}=0\), whereas the total specific energy of the lens cap’s initial orbit, \(-GM_{\oplus}/2a\) is augmented by \(\frac{1}{2}v_{i}^{2}\). Given the new semi-major axis, \(a_{\rm new}\) and the before-and-after conservation of \(h=(GM_{\oplus}a_{\rm new}(1-e^2))^{1/2}\), one can solve for \(e\), and then proceed to all four orbital elements by noting that \(r=a(1-e\cos E)\) and \(M=E-e\sin E\), and then working out the longitude of pericenter, \(\varpi\), relative to the reference direction defined by the radius vector from the Earth’s center to the point where the lens cap was thrown. Clumsy.
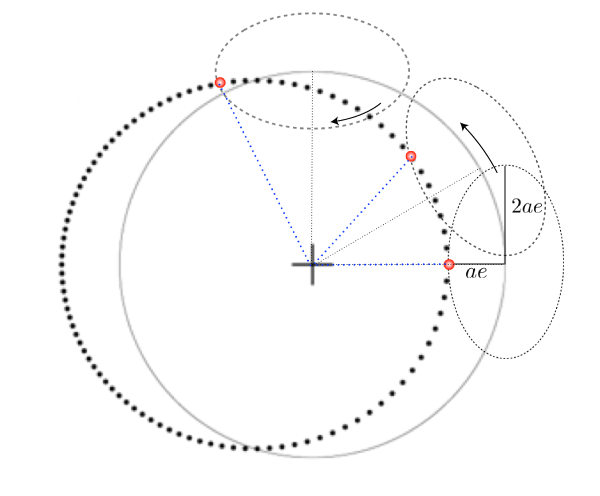
The guiding center approximation revives the old idea of epicycles to describe the motion of a particle (in this case, the lens cap) on a low eccentricity orbit. For modest \(e\), the true Keplerian motion is approximated as a compound of the circular motion of a “guiding center” and the counter-directed motion about the guiding center on a 2:1 ellipse, where the semi-minor axis is oriented radially, and has length \(ae\). Both motions complete once per orbital period. Here’s the basic idea, drawn for an orbit with \(e=0.3\), which is actually quite a substantial eccentricity:
For the lens cap problem, the guiding center materializes at a distance \(2ae\) ahead of the launch point. The motion associated with the guiding center is the superposition of two simple harmonic motions. For the radial oscillation, \(\frac{1}{2}v_{i}^{2}=\frac{1}{2}n^{2}x^{2}\rightarrow v_{i}=nae\), which works out to \(e=0.0013\). To first (and very good) approximation, the lens cap arrives back 88 minutes later in the close vicinity of the spacecraft, after inward and outward radial excursions of 8.4 km, and after leading the spacecraft by as much as \(4ae=34\) km. The small total gain in orbital energy lengthens the lens cap’s orbital period slightly, which means that the cap fails to catch up by a few tens of meters at the end of a full one-orbit epicyclic oscillation.
In Michael Rowan-Robinson’s Cosmology (3rd ed.) Oxford University Press. pp. 62–63, one finds, “It is evident that in the post-Copernican era of human history, no well-informed and rational person can imagine that Earth occupies a unique position in the universe.”
If one insists on a strictly inertial frame, I guess that’s true, but non-inertial frames often have more value. Thousands of extrasolar planets have been found, and not one of them is remotely habitable. Many lines of evidence are beginning to point toward an Earth that is unique, probably in the galaxy, and perhaps, even, in the accessible universe. In the post-post Copernican era, epicycles (2:1, rather than 1:1) have a certain appeal. And indeed, there’s nothing inherently wrong about the Tychonic model of the solar system, it simply subscribes to an Earth-centered point of view. Don’t we all?
On the topic of epicycles, I have to say I wasn’t a fan of the article on “retrograde beliefs” that appeared a few weeks ago in the New York Times Magazine. Obviously, astrology is a bunch of bunk. It’s known to be wrong. One can make the argument that taking it down in the genteel and informed confines of the NYT magazine amounts to shooting fish in a barrel. Satire is probably the best approach. Aside from this stylistic quibble, the writing in the NYT piece seems incoherent, somehow second-hand and artless. An intricate 1756 diagram by James Ferguson (who is no longer around to defend his work) is given a rather underhanded misrepresentation:
The full title of Ferguson’s book is Astronomy Explained Upon Sir Isaac Newton’s Principles, And Made Easy to Those who Have Not Studied Mathematics. The diagram reproduced in the NYT is not the product of some recalcitrant Ptolemaic view as implied in the caption, but rather appears in a chapter entitled “The Phenomena of the Heavens as seen from Different Parts of the Solar System”. It shows the intricate motions of the inferior planets in a co-rotating Earth-centered frame, and Ferguson gives a fascinating description of the analog-computational method he used to create the diagram: