The minimum threshold level for amazement will rise quickly once Kepler’s discoveries start to accumulate, and already, it’s getting very hard to remember which transiting planet is unusual for which reason. Let’s see, was it TrES-4 or WASP-17 that had that styrofoam-like density? Or was it both of them?

Even in a crowded field, though, HAT-P-13 is likely to endure as a touchstone. In the next five years, it’s likely that there will emerge only a select handful of systems in which a well-characterized transiting planet orbiting a relatively bright star is being substantially perturbed by a companion on a well-characterized orbit:

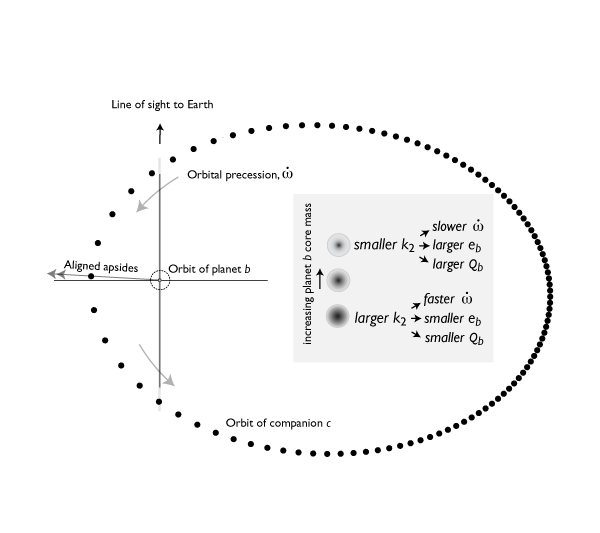
After the HAT-13 system was announced, we showed that the planets “b” and “c” should have evolved to an eccentricity fixed point configuration, in which the orbits’ apsidal lines co-rotate, and in which the orbital eccentricity of planet “b” has a very sensitive dependence on its internal structure. Further modeling, using reasonable assumptions, gives strong limits on the tidal Q of planet “b”. In essence, one can potentially accomplish with an exoplanet a big chunk of what the Juno Mission expects to accomplish at Jupiter at of order a thousandth of the cost.

Our analysis assumed that HAT-P-13 b and c are on co-planar orbits. There’s an interesting new paper by Rosemary Mardling that explores the significantly more complex situation that arises if the orbital planes of the planets are significantly misaligned. In this case, tidal dissipation in the inner planet causes the system to settle into a limit cycle, where the eccentricity and the angle between the apsides circulate on a secular timescale, and the easy insight into the structure of planet b is no longer possible.

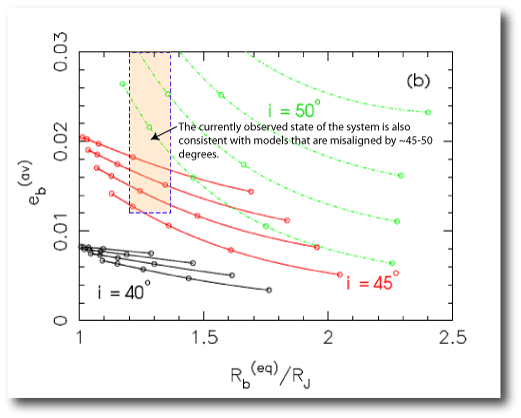
Interestingly, however, Mardling’s analysis suggests that if the orbits are misaligned, then the mutual inclination is likely to be in the neighborhood of 45 or 50 degrees. A mutual inclination of, say, 30 degrees is inconsistent with the currently observed parameters of planet b. The following two diagrams (figure 8 a and b) from her paper show how this works:

Within the next few months, we should get improved values for the eccentricity and radius of planet b, which will significantly shrink the size of the peach-colored boxes in the two figures above. HAT-P-13c is also currently headed in for periastron, with the next transit opportunity scheduled for April 12, 2010. A transit by planet c would provide strong evidence that the system is reasonably close to co-planar (and would be quite remarkable in its own right!) Furthermore, during the periastron passage of c, there should be readily detectable transit timing variations for b, which should give us a shot at distinguishing between the co-planar case and the case with a mutual eccentricity of 45-50 degrees. In the next post, I’ll look in detail at the numbers…